New Zealand Trialling Centimetre Level GNSS
Date: September 7, 2017
Since the Global Positing System (GPS) was launched by the United States in the late 1970s, modern civilisation has developed an ever increasing dependence on global navigation systems. Think of the many GPS equipped devices and applications which we use on a daily basis, such as modern cars and smart phones with applications like turn by turn navigation and everyone’s favourite phone app – Pokémon go! Such applications do not require high levels of positional accuracy and therefore can utilize a single satellite receiver. However, numerous industrial applications such as land surveying, precision agriculture as well as emerging fields like precision drone control and autonomous driving, all demand much higher levels of positioning accuracy than what has been achievable by single receiver positioning in the past.
For this reason (among others) Land Information New Zealand (LINZ) announced in 2016 that it was collaborating with Geoscience Australia in a two year trial of some of the latest global navigation technologies - known as Satellite Based Augmented System (SBAS) and Precise Point Position (PPP).
Before we take a look into these new technologies we will review the methods which are presently used to enhance the positional accuracy of Global Navigation Satellite Systems (GNSS). This will highlight the advantages these new technologies will bring and see why these new technologies could open a number of new product avenues.
Standard Single Receiver Positioning
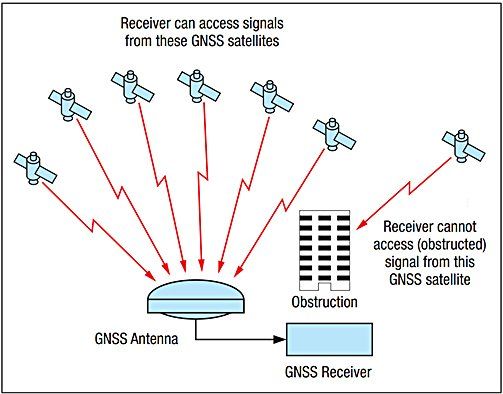
Many readers will be aware of the basics of standard GNSS positioning, but we will briefly recap this. GNSS consist of over 80 orbiting satellites (which includes GPS (American System), GLONASS (Russian system), Galileo (European System) and BeiDou (Chinese system)), the key components of these satellites are, extremely accurate atomic clocks and multiple transmitters. To allow a GNSS receiver (ground based device) to be able to calculate its position, it needs to know exactly where each satellite is in space at the precise time the GNSS signals are broadcasted from each satellite. For this reason, each satellite transmits among other things, time (at which the signal is sent), date, satellite health (whether it is currently in operation) and a unique ID known as the Pseudo-random Code Number (PRN). Since the speed at which the signal propagates through the atmosphere is known, the receiver can calculate the distance to that satellite. With a minimum of four viewable satellites and using both the known position of the satellites in space as well as the distance to each of the satellites, the receiver can resolve its position anywhere on earth using trilateration. A simple diagram of this can be seen below – Figure 1.
Figure 1 – GNSS receiver with GNSS satellites transmitting their information needed for receiver to calculate its position [2]
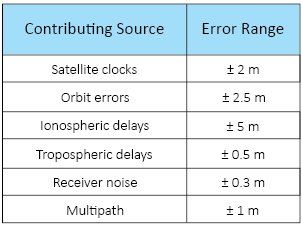
Whilst this system is effective and extremely powerful, allowing a single receiver to calculate its position anywhere on earth (assuming line of sight to satellite) there are significant positional errors, ranging from 1 – 10 m. These are largely due to the following effects: satellite clock drift, satellite orbital error and atmospheric effects from both the Ionosphere and troposphere with both affecting the signal propagation to the receiver. See the table below showing the contribution of these errors.
Figure 2 – Contributing factors to GNSS positional error [2]
Relative Position Correction or Differential GNSS (DGNSS)
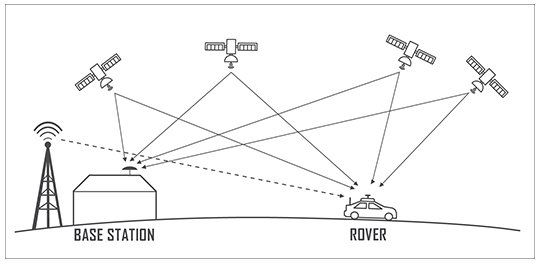
Due to the errors associated with a single receiver (as outlined above), many industries have adopted methods of eliminating or reducing these errors. One of the common methods which was developed to overcome these downsides is known as Differential GNSS (DGNSS). DGNSS typically works by having a reference station in a known fixed location. The reference station includes both a GNSS receiver and some form of radio link (such as 433 MHz or GSM) connecting the reference station to the “rover”. Since the reference station has a known location any difference in the calculated position and its actual position can be considered as an error. This error or difference is then sent out to the rover via the radio link, allowing for the error to be subtracted at the rover – see illustration below. The typical accuracy using such a system will usually be around 1 - 2 m.
Note: This error is largely attributed to the system being unable to resolve higher levels of accuracy due to the inherently low resolution/frequency of the GNSS “binary code phase”.
Figure 3 – Diagram showing key features of DGNSS
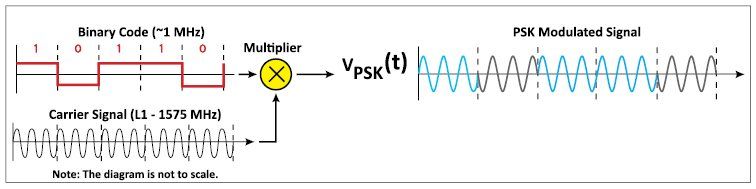
To further improve the positional accuracy, a method of Real Time Kinematic (RTK) was developed in the mid-90s. Like DGNSS, RTK also makes use of a reference station to send correction data out to the rover. However, in essence this system overcomes the error introduced by low frequency/resolution of the binary code phase (~1Mhz), by utilizing the much higher carrier phase frequency (1575 MHz) to calculate its distance to a satellite – see Figure 4. This results in a substantial improvement, typically resulting in positional accuracy of 1 - 2 cm.
Figure 4 – Signal modulation used by GNSS satellites – note carrier wave is convolved with Binary Code to generate modulated signal
There are however a number of draw backs to these systems, not least the requirement of the local infrastructure, where a local reference station is required. This drives up the installation, product and maintenance cost, with traditional RTK systems (including receivers and reference stations) having been in the tens of thousands of dollars. For this reason, the applications have been limited to high value applications such as cropping tractors and surveying equipment. In addition to the high infrastructure cost, RTK also has limitations to its baseline (the distance between the rover and the reference station) – which is typically limited to 20 - 30 km. The limited “baseline” distance is a due the varying atmospheric (ionospheric and tropospheric) effects - which the reference station is accounting for - eventually have little correlation to what the rover is observing.
Satellite Base Augmented System (SBAS)
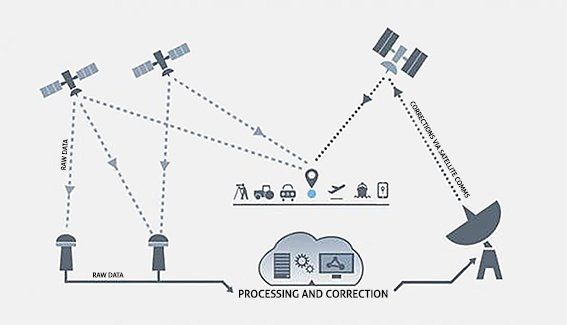
Much like DGNSS, the Satellite Based Augmented System (SBAS) also makes use of reference stations to provide correction data to the rover. However, rather than using a single reference station, SBAS makes use of several reference stations spread over the entire operational area (New Zealand’s trial makes use of 5 stations). By using several reference stations a map of the atmospheric effects is generated and this allows the system to cover large areas. Along with the atmospheric effects, the reference stations also allow for correction data of satellite orbital error and satellite clock errors to be accounted for. The correction information is then beamed to a geostationary satellite, which in turn broadcasts the correction data back to earth, allowing the rover to make a local correction. Since this correction data is sent over the existing GPS L1 channel (the original civilian channel - see figure 6) this can be received by existing GNSS receivers without the need for any additional antennas or hardware. This means that the existing single receiver GNSS devices could make use of this correction data with just a firmware update. Systems similar to SBAS have been available in the USA (Wide Area Augmentation System WAAS) and Europe for a number of years and allows for a positional accuracy of 1 - 2 m
Figure 5 – Diagram of SBAS core features [5]
In addition to standard SBAS, the LINZ and Australian Geoscience trial will also be testing the second generation SBAS system which uses the latest Dual Frequency Multi Constellation (DFMC) setup. DFMC uses both the GPS L1 and the most recently added L5 channel (see figure 6), by making use of both these channels it is expected to reduce the positional error to less than 1 m (the exact error is still unknown as this will be the first trial of its kind). Whilst the standard SBAS has been available in New Zealand since the 1st of June 2017, the SBAS DFMC trial is forecasted to start later this year. However, due to this being the first SBAS DFMC trial, there is presently no “Off the Shelf” hardware available to support it, furthermore there are still only a handful of satellites which support the GPS L5 channel and therefore may have limited use initially.
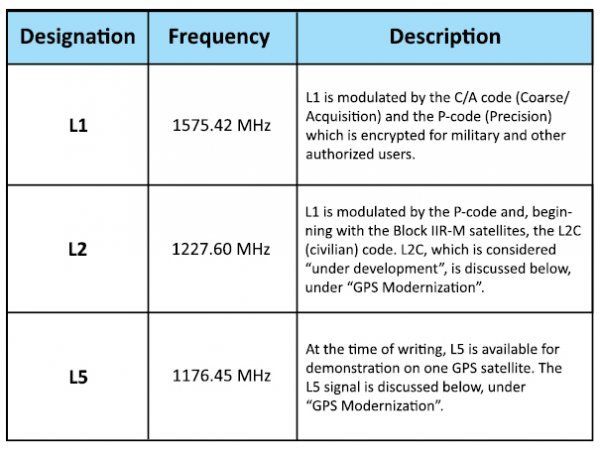
Figure 6 – Present GPS channels [2]
Precise Point Positioning PPP
Precise Point Positioning is an exciting technology which can achieve positional accuracy similar to RTK but using just a single receiver. Although there are no strict standards on PPP operation, the principal of PPP is essentially to use extremely precise satellite clock and orbital correction data which is collected and processed through a number of reference stations positioned globally (rather than locally for SBAS). The satellite correction data for the New Zealand trial will be broadcast along with the SBAS data over the L1, L2 and L5 channels. Along with the precision satellite correction data, dual frequency PPP devices takes advantage of the fact that ionospheric propagation delays are directly proportional to the frequency of the signal. This means that by using a dual frequency receiver, i.e. reading both L1, L2 and L5 (see figure 6,) the receiver has the ability to almost entirely remove the ionospheric errors.
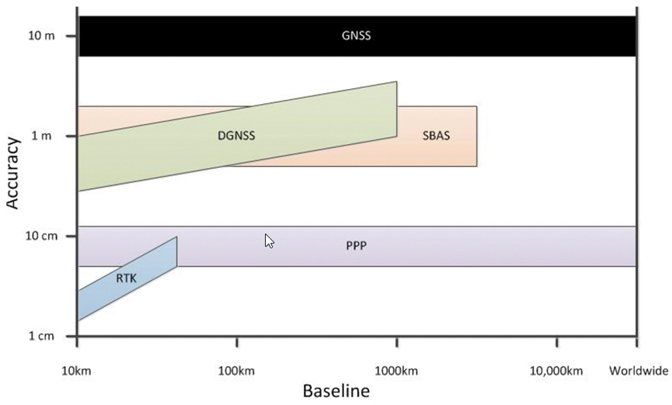
The big advantage of dual frequency PPP is that its baseline (operational area) is global, this is because its atmospheric adjustments are no longer based on local reference stations but rather frequency dependent. Of the four positional technologies discussed, PPP shows the best baseline vs accuracy - see Figure 7 below. However, the drawback of PPP is the long convergence time (time to gain an accurate initial positional reading). This convergence time can be anywhere from 20 minutes up to several hours and this therefore makes PPP (on its own) less attractive in real time applications.
Figure 7 - Positional accuracy vs Baseline of different GNSS correction methods [9]
Conclusion
With the combined technology of both PPP and SBAS, the positional accuracy is projected to be around 5 cm for dual frequency (L1 and L5) single GNSS receivers and sub meter accuracy for low cost single frequency GNSS receivers, such as Ublox NEO-7P. Whilst positional systems providing 5 cm or greater positional accuracy has been available for the last 20 years through the use of RTK systems, the cost of such systems have always made it uneconomical for a number of applications, with the infrastructural cost of such system being in the tens of thousands of dollars. The end effect is that by using SBAS and PPP extremely accurate positioning can now be achieved at a fraction of both the product design and manufacturing costs. Furthermore, unlike local DGPS or RTK systems, the onus of the infrastructural cost and maintenance is no longer with the consumer or product user, but rather with government. For these reasons, this looks to be an extremely exciting technology which could have big implications in a number of industries such as Unmanned Aerial Vehicles (UAV), autonomous cars, precision agriculture and surveying.
Although the SBAS and PPP services are still in their trial phase and will likely end early 2019, we at Beta Solutions are excited about the future such technologies pose. These technologies will allow us to further enhance our GNSS product design solutions and will give our clients a leading edge in their respective industries, thus we will be following the developments of this trial closely. If you’d like to find out more about how SBAS or PPP enhanced GNSS could benefit your business, please do get in touch via our contact page.
References:
- u-blox, “Precise Point Positioning (PPP),”
- Jeffrey, C., (NovAtel, 2010). “An Introduction to GNSS.”
- Vlajoc, N., (2010). “Analog Transmission Analog Transmission of Digital Data: of Digital Data: ASK, FSK, PSK, QAM ASK, FSK, PSK, QAM.”
- Alkan, R.M., Ozulu1, I.M. and İlçi1, V., (May, 2016). “Precise Point Positioning (PPP) Technique versus Network-RTK GNSS.”
- CRCSI, (July, 2017). “Technical Specifications Document for Satellite-Based Augmentation System (SBAS) Testbed.”
- NovAtel. “About PPP.”
- ESA Nauipedia, (2011). “RTK Fundamentals”
- ESA Nauipedia, (2011). “PPP Standards”
- NovAtel. “GNSS Data Post-Processing.”